020 Dozenapentic (60) Introduction

3 x 4 x 5 = 60.
60 is divisible by 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30, 60.
Under Construction
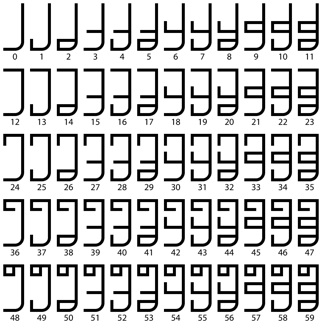
Here we see a glyph system that starts out cycling two lines at the bottom, then three lines in the middle, then four lines at the top. This gives us sixty combinations, five sets, of four sets, of three sets.
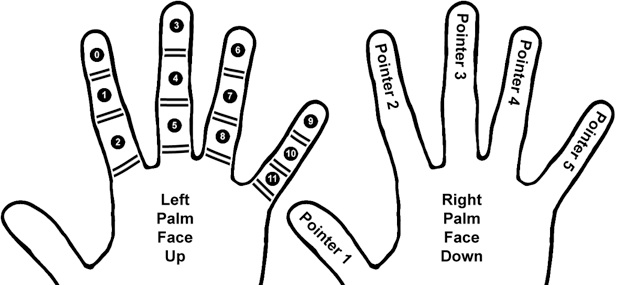
Using both hands, it’s easy to count in base sixty. It’s also easy to add. To add two glyphs together, we first locate it on our hands. The upper portion of the glyph tells us which finger to use as a pointer. The middle part of the glyph tells us which finger to point to. The lower part of the glyph refers to the knuckle that is pointed to. At this point, it’s easy to add a second glyph to the first. We break the second glyph down into it’s three parts. These parts are then added individually. The upper part of the second glyph determines which pointer finger to use, the middle determines which finger to move to, and the lower determines which knuckle to move to. If you run out of knuckles or fingers, you carry up to the the next part of the glyph, or to the next glyph in the positional notation system.

There are other ways to represent base sixty. Here we see a glyph system that starts out cycling five lines at the bottom, then four lines in the middle, then two lines at the top. This is a reversal of the previous system. I suspect that it would be less useful, though it is worth consideration.
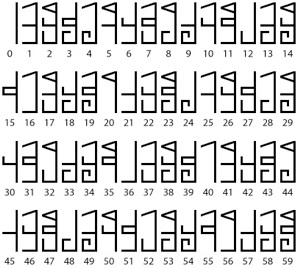
Similar to Dozenic Syncopation, it is possible to set this glyph system to a syncopated pattern as well. In this example, we see all portions of the glyph cycling individually.
This may seem unusually complex, but may prove to be useful. It would require complete memorization of the glyphs, but I suspect that there could be some interesting benefits.
I plan to experiment with this system and report my findings in future entries.

