Numerography

This website blog chronicles my exploration into using symbology to represent alternative numerical base systems. My ultimate goal is to uncover and simplify known, and unknown, mathematical processes and connections between these systems. This is a hobby and should be taken as such. Thanks for visiting.

017 Dozenic Finger Counting

2006-05-13 Filed in: Dozenic
How does a person count in base twelve using only one hand? It turns out, there IS an easy way to do it.



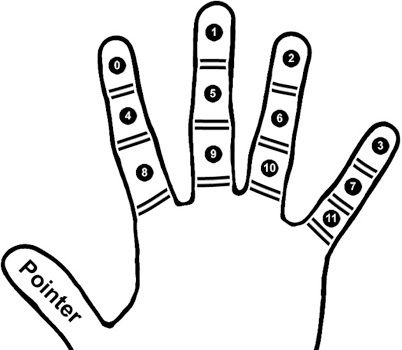
doz_palm_2
Hold your left palm open and facing toward you. The idea is to use your thumb as a pointer by tapping on the individual phalanx bones in the remaining four fingers. Each finger contains three phalanges. That makes twelve tapping areas.

Most aspects of finger counting apply. Instead of using individual fingers to represent integers, you are using sections of the fingers.

This same system can be mirrored on the opposing hand as well. When we run out of phalanges on the left hand, we can simply point to a new right hand phalanx with our right thumb. Each right hand phalanx equals one dozen. The right hand should be treated as a new digit that uses a base twelve numerical positioning system.

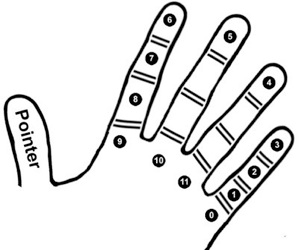
doz_palm_3



This then provides an easy system for adding/subtracting double digit dozenic numbers. In decimal, that translates to a range of 0 to 144.

I created an alternative method of finger counting that might work better for Dial Dozenic users. If the hand is held slightly rotated, as in the above example, you can trace out a diamond shaped pattern of tapping areas that coincides with the symbolism used in Dial Dozenic. This system not only uses phalanges, but also uses the four callous covered joints on the palm at the base of the fingers.



doz_palm_4


I’ve also been experimenting with syncopated patterns of counting. The above example tries to mimic the symbology used in Syncopated Dozenic. To count, one would tap phalanx in a diagonal direction. When you run out of phalanges in that direction, counting would continue on the flipped side of the hand. The process would ultimately become easier as soon as a person commits the positions and sequence to memory.






This may seem strange and unnecessarily complex at first, but it may turn out that this alternative pattern might aid in more complex computational procedures. I plan to keep researching and comparing all these systems, as well as other similar variations that are not listed here.

Tags: ,